Long Division of Polynomials
Divide:
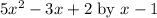
Arranging dividend and divisor for the long division procedure:r thefrrrArA
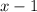
Divide the first term of the dividend by the first term of the divisor:of the d
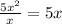
![\begin{gathered} \text{ }5x \\ x-1\text{ \mid }5x^2-3x+2 \end{gathered}]()
Multiply 5x by the divisor: div
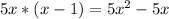
Subtract this product from the dividend:
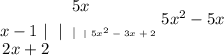
Now divide 2x+2 by x = 2. Repeat the procedure:rocedurrocedu
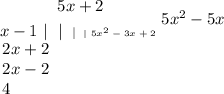
The quotient is 5x + 2 and the remainder is 4
a