1) y = (x -4)(x -5)
2) vertex (4.5, -0.25)
x value = 4.5
3) x intercept: 4 and 5
y intercept: 20
Step-by-step explanation:
Part 1:
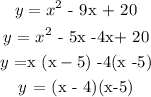
Graphing the equation:
The equation in factored form:
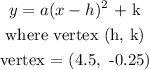
Part 2:
The vertex of the parabola is (4.5, -0.25)
The x value of the vertex:
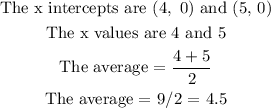
Part 3:
The x intercept is the point the y value is zero
y = x² - 9x + 20
0 = x² - 9x + 20
0 = (x - 4)(x -5)
(x -4) = 0 or (x - 5) = 0
x = 4 or x = 5
The x intercepts are 4 and 5
In ordered form: (4, 0) and (5, 0)
we were asked to se the equation:
The y intercept is the point the x value is zero
y = (0)² -9(0) + 20
y = 0 - 0 + 20
y = 20
the y-intercept is y = 20
In orderd form: (0, 20)