Question

Amanda and Mofor are selling wrapping paper for a school fundraiser. Customers can buy rolls of plain wrapping paper and rolls of holiday wrapping paper. Amanda sold 13 rolls of plain wrapping paper and 12 rolls of holiday wrapping paper for a total of $208. Mofor sold 4 rolls of plain wrapping paper and 3 rolls of holiday wrapping paper for a total of $55. Fine the cost of one roll of plain wrapping paper and one roll of holiday wrapping paper.

Answer 1

Given:

Amanda sold 13 rolls of plain wrapping paper and 12 rolls of holiday wrapping paper for a total of $208.

And,

Mofor sold 4 rolls of plain wrapping paper and 3 rolls of holiday wrapping paper for a total of $55.

Let, x be the cost of one roll of plain wrapping paper and y be the cost of one roll of holiday wrapping paper.

The equations are,

`\begin{gathered} 13x+12y=208\ldots\ldots\ldots\text{.....}(1) \\ 4x+3y=55\ldots\ldots..\ldots\ldots\ldots\text{.}(2) \end{gathered}`

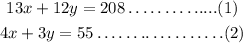
Solve the equations,

`\begin{gathered} 4x+3y=55 \\ 4x=55-3y \\ x=(55-3y)/(4) \\ \text{Put it in quation (1)} \\ 13x+12y=208 \\ 13((55-3y)/(4))+12y=208 \\ (715-39y)/(4)+12y=208 \\ 715-39y+4(12y)=4(208) \\ 715-39y+48y=832 \\ 9y=832-715 \\ 9y=117 \\ y=(117)/(9) \\ y=13 \end{gathered}`

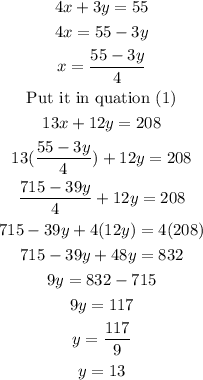
Put the value of y in equation (2),

`\begin{gathered} 4x+3y=55 \\ 4x+3(13)=55 \\ 4x+39=55 \\ 4x=55-39 \\ 4x=16 \\ x=(16)/(4) \\ x=4 \end{gathered}`

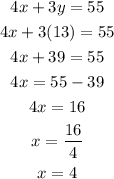
Answer:

The cost of one roll of plain wrapping paper is x = $4.

The cost of one roll of holiday wrapping paper is y = $13.