Question

Imagine you are working for Hasbro making Gummy Bear containers. On a day to day basis you fillup two different size containers with gummy bears. One of the containers is 3.0 x 5.7 x 6.9 indimensions and contains 316 gummy bears. The other is 8.3x7.7 x 8.7 in dimensions. About howmany gummy bears would fit in the box? Round to the nearest whole number.

Answer 1

For the first container, we have a volume of:

`3.0\cdot5.7\cdot6.9=117.99`

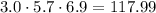
That is, 117.99 in³.

For the second container, we have a volume of:

`8.3\cdot7.7\cdot8.7=556.017`

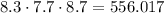
Each Guumy Bear has a volume, thus they represent a volume.

So, the ratio of the volumes of the container must be the same as the ratio of how many Gummy Bear fit in each container.

The ratio of the first and the second box is:

`(117.99)/(556.017)`

And, if we call the number of Gummy Bear that fits the second container "x", the ratio of the number f Gummy Bear is:

`(316)/(x)`

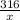
Since these ratios must be equal:

`\begin{gathered} (117.99)/(556.017)=(316)/(x) \\ 117.99x=556.017\cdot316 \\ x=(556.017\cdot316)/(117.99)=1489.12\ldots\approx1489 \end{gathered}`

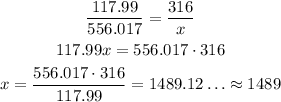
Thus, about 1489 Gummy Bears would fit the the second container.