Answer:
• 1st zero: x=-2/3 with a multiplicity of 5.
,
• 2nd zero: x=5 with a multiplicity of 2.
Explanation:
Given the function:
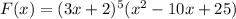
First, factorize the quadratic expression: x²-10x+25
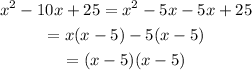
Therefore, we can rewrite F(x) as:
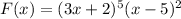
Solving for the zeroes:
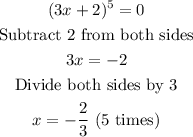
• 1st zero: x=-2/3 with a multiplicity of 5.
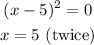
• 2nd zero: x=5 with a multiplicity of 2.