Step-by-step explanation:
The inequality theorem states that the sum of any two sides of a triangle must be grater than the sum of the third side.
We have to try this rule for each option:
• {15, 27, 44}
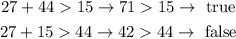
This cannot represent the sides of a triangle.
• {10, 24, 33}
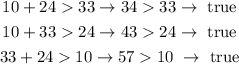
This set can represent the sides of a triangle.
• {5, 19, 25}
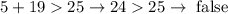
This set cannot represent the sides of a triangle.
• {6, 12, 19}
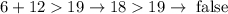
This set cannot represent the sides of a triangle.
Answer:
The set of numbers that could reprensent the sides of a triangle is {10, 24, 33}