Answer:
The sample mean is of 1970 daily calories.
The margin of error is of 120 daily calories.
The sample standard deviation is of 12.3 daily calories.
Explanation:
Mean number of daily calories consumed by participants in a medical study is (1850, 2090).
The sample mean is the mean of the bounds of the confidence interval. So
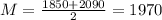
The sample mean is of 1970 daily calories.
Margin of error:
The margin of error is the difference between the bounds and the sample mean. So
2090 - 1970 = 1970 - 1850 = 120
The margin of error is of 120 daily calories.
Sample standard deviation:
Now I have to expand a bit into the t-distribution. So
The first step to solve this problem is finding how many degrees of freedom, we have. This is the sample size subtracted by 1. So
df = 22 - 1 = 21
95% confidence interval
Now, we have to find a value of T, which is found looking at the t table, with 21 degrees of freedom(y-axis) and a confidence level of
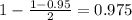 . So we have T = 2.08
. So we have T = 2.08
The margin of error is:

In which s is the standard deviation of the sample.
Since
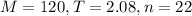
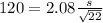
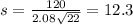
The sample standard deviation is of 12.3 calories.