Question

Find the equation of the line(s) through (2,-2) if the sun 10. Find the angle that line 7, makes with line l.. -11. Find the coordinates of the point. * Find the value ota itx (a) + = 10, 1,: 3x - 2y = 5 (b) . (a) equidistant from (4.-) and the origin; as well as o (b) equidistant from (3.8) and (-2,5) on the y-axis (e)

Answer 1

We are asked to determine the coordinates of a point that is equidistance to the point (4, -6) and the origin. To do that we need first to determine the distance of this point to the origin using the following formula:

`d=\sqrt[]{(x-x_0)^2+(y-y_0)^2_{}}`

Since the origin has coordiante (0, 0), this means:

`(x_0,y_0)=(0,0)`

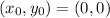
Replacing:

`d=\sqrt[]{(x)^2+(y)^2_{}}`

Now, replacing the point we get:

`d=\sqrt[]{(4)^2+(-6)^2_{}}`

Solving the operations we get: