The system of equations is:

To solve by substitution, first we need to isolate one of the variables. However, this is already done in the first equation.
Next, we want to substitute x into the other equation, so let's do that:

Notice that we divide both sides by two to simplify our work:

Now we isolate y:
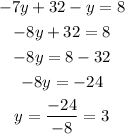
Now that we have y, we substitute in either equations to find x. Let's do it in the first one:

So, y = 3 and x = 11.