Answer:
The valid formula for the surface area are;

Step-by-step explanation:
Given the regular pyramid with;

The total surface area is the sum of the Base area and the Lateral area.
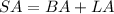
And the formula for calculating the lateral area of the pyramid is;

substituting LA into the formula above;

The valid formula for the surface area are;
