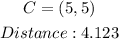Given:
There are given that the coordinates:

Step-by-step explanation:
First, we need to find the mid-point of c by using the given coordinate point A and B.
Then,
To find the midpoint, we need to use the midpoint:
So,
Fro the formula of midpoint:
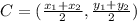
Where,
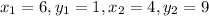
Then,
Put all the values into the above formula:
So,
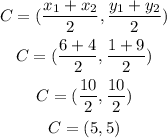
So,
The point of C is, (5, 5).
Now,
We need to find the distance between A(6,1)and C(5,5):
So,
From the distance formula:
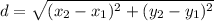
Where,
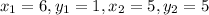
Then,
Put all values into the above formula:
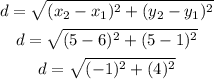
Then,

Final answer:
Hence, the midpoint C and distance of A and C is shown below: