Question

I’m supposed to be finding where the two lines intersect (x,y)Y= -5x+13 & Y= -x+7

Answer 1

We can find where the two lines intercept by finding the solution of the system of equations given

y=-5x+13

y=-x+7

Because the equations are equalities of y we can do the next equality

`-5x+13=-x+7`

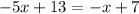
we simplify

`-5x+x=7-13`
`-4x=-6`

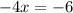
Then we isolate the x

`x=(-6)/(-4)=(6)/(4)=(3)/(2)`

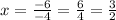
And then we use the first equation in order to find y we substitute the value of x

`y=-5((3)/(2))+13=(11)/(2)`

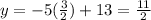
Therefore the point where the two lines intersect is (3/2,11/2)