The equation:
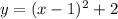
has the form:
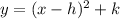
where the point (h, k) is the vertex of the parabola. In this case, the vertex is located at (1, 2).
The axis of symmetry is
x = h
x = 1
To graph the function we need three points. One of them is the vertex. The other two should be at the same distance from the axis of symmetry. Substituting with x = 0 into the equation, we get:
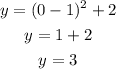
Substituting with x = 2 into the equation, we get:
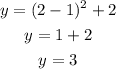
Connecting with a parabola the points (0, 3), (1, 2), and (2, 3) we get: