Question

2. Your municipality is replacing the storage tanks in the community. Which plan provides the greatertotal capacity?Plan 1: Install one cylindrical tank that is 150 feet tall and has a radius of 50 feet.Plan 2: Install two cylindrical tanks that are 75 feet tall. One cylindrical tank has a radius of 30 feet,and one tank has a radius of 25 feet.Use 3.14 for π. Round your answers to the nearest tenth, if necessary.

Answer 1

To find which plan provides the greater total capacity, we will find the volume of each plan.

The storage tanks have a cylindrical shape.

The cylinder of a base radius of r and height of h has a volume of V

`V=\pi\cdot r^2\cdot h`

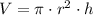
Plan 1: Install one cylindrical tank that is 150 feet tall and has a radius of 50 feet.

`\begin{gathered} h=150\text{ ft} \\ r=50\text{ ft} \\ V=3.14\cdot50^2\cdot150=1,177,500\text{ ft\textasciicircum{}3} \end{gathered}`

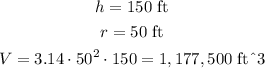
Plan 2: Install two cylindrical tanks that are 75 feet tall. One cylindrical tank has a radius of 30 feet, and one tank has a radius of 25 feet.

`\begin{gathered} h=75\text{ ft} \\ r_1=30\text{ ft} \\ r_2=25\text{ ft} \\ V=3.14\cdot75\cdot(30^2+25^2)=359,137.5\text{ ft\textasciicircum{}3} \end{gathered}`

So, by comparing the volume of the planes

The volume of plan 1 > the volume of plan 2

So, the answer will be plan 1 will provide the greater total capacity.