Question

Solve the equations by dividing the left-hand side by the multipliers according to the difference of squares formula: (e) 3x^2 - 10 = 0

Answer 1

Given:

There is a equation given in the question

`3x^2-10=0`

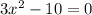
Required:

Solve equation by difference of squares formula.

Step-by-step explanation:

First of all divide left side with 3

`\begin{gathered} (3x^2-10)/(3)=0 \\ x^2-(10)/(3)=0 \end{gathered}`

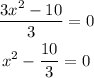
We have formula for difference of squares

`a^2-b^2=(a-b)(a+b)`

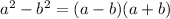
as formula

`\begin{gathered} x^(2)-(10)/(3)=0 \\ (x-\sqrt{(10)/(3)})(x+\sqrt{(10)/(3)}_)=0 \\ x=\pm\sqrt{(10)/(3)} \end{gathered}`

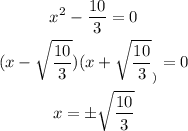
Final answer:

Solution of given equation is