Given
The equation is given as
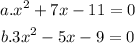
Step-by-step explanation
a. The equation is given as
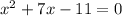
The general equation is

The sum of the root is determined as

Substitute the values from the given equation.
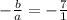
The product of roots is determined as

Substitute the values from the given equation.
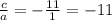
b. The equation is given
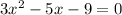
The sum of root is
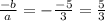
The product of root is
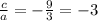
Answer
a. The sum of root is -7.
The product of root is -11.
b. The sum of root is 5/3.
The product of root is -3.