Question

At steady state, the power input of a refrigeration cycle is 500 kW. The cycle operates between hot and cold reservoirs which are at 550 K and 300 K, respectively. a) If cycle's coefficient of performance is 1.6, determine the rate of energy removed from the cold reservoir, in kW. b) Determine the minimum theoretical power required, in kW, for any such cycle operating between 550 K and 300 K

Answer 1

The answer is below

Step-by-step explanation:

Given that:

Hot reservoir temperature (
`T_H`) = 550 K, Cold reservoir temperature (
`T_C`) = 300 K, power input (
`W_(cycle)=500 \ kW`), cycle's coefficient of performance(
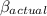`\beta_(actual)`) = 1.6

a) The rate of energy removal in the cold reservoir (
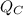`Q_C`) is given by the formula:

`Q_C=\beta_(actual)* W_(cycle)\\\\Q_C=1.6*500\\\\Q_C=800\ kW`

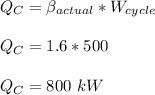
b) The maximum cycle's coefficient of performance(
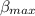`\beta_(max)`) is:

`\beta_(max)=(T_C)/(T_H-T_C)=(300)/(550-300)=1.5\\\\For\ minimum\ theoretical\ power\ \beta_(max)=\beta_(actual)=1.5\\\\W_(cycle)=(Q_C)/(\beta_(actual)) =(800)/(1.5) \\\\W_(cycle)=533.3\ kW`