The graph of an inequality whose solution is a half-plane has a boundary line and one of the regions (up or below) the line is shaded. This shaded region corresponds to the solution set of the inequality.
When the boundary line is solid, i.e., is included in the solution set, we say that the half-plane is closed.
When the boundary line is dashed, i.e., not included in the solution set, we say that the half-plane is open.
In this problem, we need to analyze inequalities with ≤ or ≥. Those symbols mean:
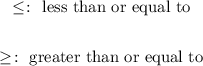
Notice that the part "equal to" is responsible for including the boundary line in the solution set.
Thus, the graph of an inequality with ≤ or ≥ is a closed half-plane.
Therefore, the statement "the graph of an inequality with ≤ or ≥ is an open half-plane" is FALSE.