Step-by-step explanation:
If Calvin starts with $1,000 per week or more, he will gain $52,000 per year or more, because:
52*$1000 = 52,000
Then, we need to standardize 52,000 with the mean and standard deviation of both agencies.
To standardize 52,000 we can use the following equation:
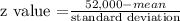
So, the z value for the first agency is:
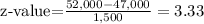
For the second agency:
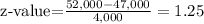
It is more probable to have a salary that is near to the mean, and the z-value gives us how far is the 52,000 from the mean.
Since the second agency has a smaller z-value, it is more likely to start with an offer of $1000 in the second agency.
Answer: The second agency