Question

Use the number line below, where RS=8y+2,ST=5y+7,and RT=113 What is the value of RS

Answer 1

The given problem can be exemplified in the following diagram:

From the line we get the following relationship:

`RS+ST=RT`

Replacing the values:

`(8y+2)+(5y+7)=113`

Associating like terms, that is, terms that have the same variable elevated to the same exponent:

`(8y+5y)+(2+7)=113`

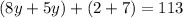
Adding like terms:

`13y+9=113`

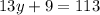
Now we subtract 9 to both sides:

`\begin{gathered} 13y+9-9=113-9 \\ 13y=104 \end{gathered}`

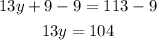
Dividing both sides by 13

`y=(104)/(13)=8`

Now we replace the value of "y" in the expression for RS:

`\begin{gathered} RS=8y+2 \\ RS=8(8)+2 \\ RS=64+2 \\ RS=66 \end{gathered}`

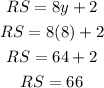
Therefore, RS is 66.