Question

HELP!!! LOTS OF POINTS AND I GIVE BRIANLEST NO FAKE ANSWERSPart A: Solve the equation 7x + 3x − 18 = 6(x + 3), and show your work. (5 points)Part B: Use this list of properties to justify each step of your math work. Justifications may be used more than once if needed. (5 points)Distributive PropertySubtraction Property of EqualityCombine Like TermsMultiplication Property of EqualityDivision Property of EqualityGivenAddition Property of Equality

Answer 1

Given:

`7x+3x-18=6(x+3)`

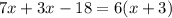
The solution will be done by performing the justifications provided as follows:

1) Given

`7x+3x-18=6(x+3)`

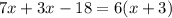
2)Distributive property or Multiplicative property of equality:

`(7+3)x-18=6x+18`

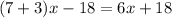
3)Addition property of equality:

`10x-18=6x+18`

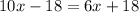
4)Combine like terms:

`10x-6x=18+18`

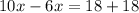
5)Distributive property and addition property of equality:

`(10-6)x=36`

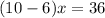
6)Subtraction property of equality:

`4x=36`

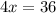
7)Division property of equality:

`\begin{gathered} x=(36)/(4) \\ x=9 \end{gathered}`

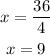
These are the steps followed and result is x = 9.