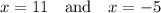We are given the following equation
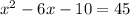
Let us solve the equation by completing the square method.
Step 1:
Move the constant term (10) to the other side of the equation.
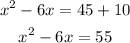
Step 2:
Add the square of half of the coefficient of the middle term (that is 6x term) to both sides of the equation
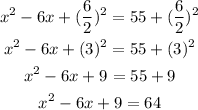
Step 3:
The terms on the left side of the equation make a perfect square

Step 4:
To solve for x, take square root on both sides of the equation
![\begin{gathered} \sqrt[]{(x-3)^2}=\sqrt[]{64} \\ x-3=\pm8 \\ x-3=8\quad \text{and}\quad x-3=-8 \\ x=3+8\quad \text{and}\quad x=3-8 \\ x=11\quad \text{and}\quad x=-5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/zlcqbz6h332122tdng5uem2jqv15j7xll4.png)
Therefore, the solution of the equation is