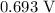Question

A 500-turn coil with an average radius of 0.06 m is placed in a uniform magnetic field so that o=40 degrees. The field increases at a rate of 0.160 T/s. What is the magnitude of the resulting emf?O0.693VO0.0014VO0.905VO0.116V

Answer 1

Given:

no. of turn in coil is

`n=500`

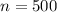
radius of the coil is

`r=0.06\text{ m}`

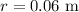
rate of change of magnetic field is

`(dB)/(dt)=0.160\text{ T/s}`

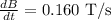
coil makes an angle with the magnetic field is

`\theta=40^(\circ)`

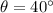
Required: magnitude of the resulting emf in the coil

Step-by-step explanation:

emf in the any coil is given by

`\epsilon=-nA(dB)/(dt)\cos40^(\circ)`

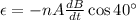
plugging all the values in the above relation, we get

`\begin{gathered} \epsilon=500*3.14*(0.06\text{ m})^2*0.160\text{ }*0.766 \\ \epsilon=0.693\text{ V} \end{gathered}`

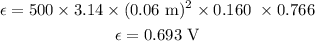
Thus, the emf in the coil is

`0.693\text{ V}`