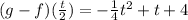Step-by-step explanation:
(g - f) means that we have to subtract f(t) from g(t)
(g - f)(t/2) means that to the resulting function from the previous operation we have to replace each t by t/2:
Let's do the first part:
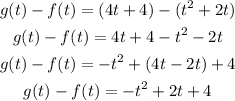
Now we have to replace t by t/2:
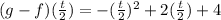
And we can simplify some fractions:
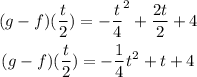
Answer: