Given:
C(0, 3) and D(0, 7)
Scale factor of dilation = 3
Lt's find the length of CD if it is dilated about point C.
To find the length, apply the formula:
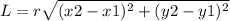
Where:
r is the scale factor = 3
L is the length of segment CD.
(x1, y1) ==> (0, 3)
(x2, y2) ==> (0, 7)
Thus, we have:

Therefore, the length of CD is 12
ANSWER:
12