Notice that the vertex of the graph of g(x) is at (0,0); therefore, no translation was applied to f(x). On the other hand, the graph of g(x) goes through (2,1) rather than (1,1); therefore, g(x) is a vertical compression of f(x).
In general, a vertical compression is given by the formula below (given a function h(x))
![h(x)\rightarrow ah(x);0Then, in our case,[tex]g(x)=a*f(x)](https://img.qammunity.org/2023/formulas/mathematics/college/ck4dxpk6j8ko3szmonccq2c9cnm9pop0r0.png)
To determine a, remember that the graph of g(x) includes the point (2,1); then,
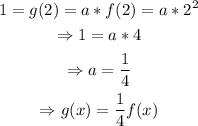
The answer is 'g(x) is a vertical compression of function f. g(x)=1/4*x^2'