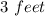Explanation
We are required to determine the maximum height of the arch model given by:
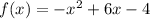
This is achieved thus:
- First, we start by determining the first derivative of the function as follows:
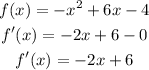
- Next, we equate the derived function to zero and solve for x as follows:

Hence, the answer is: