If we follow the construction, we get the following picture
We want to determine the position of R to fullfill the given condition. First, let x the be the distance from Q to R, and let y be the distance from R to S. At a first glance, the sum of x and y should add up to the total distance between S and Q. To calculate the distance between Q and S, we simply subtract the position of Q and the position of S. In our case the total distance is

So, in our notation, we get the equation
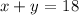
On the other hand we are told that the point R divides the segment in a 7:9 ratio. That is, the ratio of the distance from Q to R (x) and the distance from R to S (y) is 7:9. That is

We can arrange this equation as
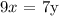
Now, consider the first equation we got (x+y=18). If we multiply both sides by 7, we get
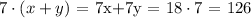
From the seconde equation, se have 7y = 9x, so if we replace this value, we get
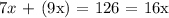
If we divide by 16 on both sides we get
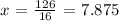
Now, since 7.875 is the distance from Q to R, we know that if we subtract the coordinates of Q and R, we should get 7.875
Then, let h be the position of R. So we have the equation
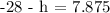
So, by adding by h on both sides and subtracting 7.875, we get
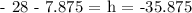
By rounding to the closest hundredth we get -35.88. So R is located at -35.88