Answer:
(144π)(30), because the area of the circular base is multiplied by the height of the cylinder to find the volume.
Step-by-step explanation:
The volume of a cylinder can be calculated as the area of the circular base multiplied by its height. So, the volume of the cylinder is:
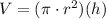
Where πr² is the area of the circular base, h is the height and r is the radius.
The radius is half of the diameter, so the radius of the circular base is:
24 cm / 2 = 12 cm
Then, the volume of the cylinder is equal to:
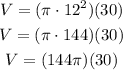
Therefore, the answer is (144π)(30), because the area of the circular base is multiplied by the height of the cylinder to find the volume.