Question

A particle moves along the x-axis so that at time t\ge 0t≥0 its position is given by x(t)=t^2+2t+12.x(t)=t2+2t+12. Determine the speed of the particle at t=4.t=4.

Answer 1

The Solution.

The given function is :

`x(t)=t^2+2t+12`

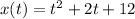
Differentiating the given function with respect to x, gives us the function that defines the speed of the particle.

`Speed=(dx(t))/(dx)=2t+2`

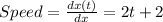
Substituting 4 for t , since the speed is at t = 4, we get

`\text{Speed =2(4)+2 = 8+2=10 units}`

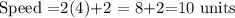
Hence, the speed of the particle at time t = 4 is 10 units (m/s)