Answer: Provided the graph of distance vs time, we have to answer (a) to (f):
(a) Student's speed from 3rd to the 5th minute:
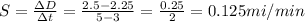
(b) At what time during the bike ride did the student come to stop:
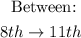
(c) During what time interval was the student's speed increasing:
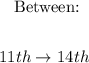
(d) What was the student's speed between 7th to 19th minute:
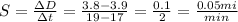
(e) During what time interval was the student's speed constant:
