Given that 1 kilogram of honeydew melon and 2 kilograms of watermelon, which cost her $7 and 3 kilograms of honeydew melon and 3 kilograms of watermelon, spending a total of $15 on the melons
Let x be the cost of a kilogram of honeydew melon and y be the cost of a kilogram of watermelon.
The system of equation is
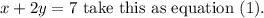
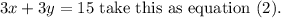
Multiply equation (1) by 3, we get
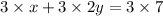
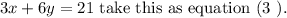
Subtracting equation (2) from equation (3), we get
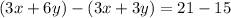

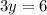
Dividing by 3, we get

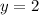
Substitute y=2 in equation (1), we get
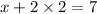
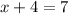
Subtracting 4 from both sides, w get
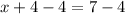
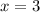
The cost of a kilogram of honeydew melon =$ 3.
The cost of a kilogram of watermelon = $ 2.