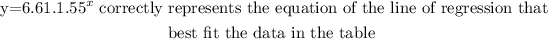Question

Question is in image.

Answer 1

The correct answer is option B

The equation of the regression line is

`\text{ y=6.6}1.1.55^x`

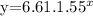
Explanations:

By substituting x into the equation above,

At x = -4, y=1.15 which is approximately close to 1.07 in the table

At x = -3, y= 1.78 which is approximately close to 1.98 in the table

At x = --2, y= 2.75 which is approximately close to 2.93 in the table

At x = -1, y= 4.26

At x = 0, y= 6.61

At x = 1, y= 10.25 which is approximately close to 10 in the table

At x = 2, y= 15.8 which is approximately close to 16 in the table

At x = 3, y= 24.61 which is approximately close to 25 in the table

At x = 4, y= 38.15 which is approximately close to 36 in the table

Since most of the points are approximately close to the values in the table,

`\begin{gathered} \text{ y=6.6}1.1.55^x\text{ correctly represents the equation of the line of regression that} \\ \text{best fit the data in the table} \end{gathered}`