Question

Graph the lines of two equations, the mark the point of intersection for the two lines. In approximately how many minutes will the two planes be at the same altitude? At what altitude will they be?

Answer 1

Given the System of Equations:

`\begin{cases}y=12-2.5x \\ \\ y=1+4x\end{cases}`

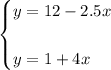
You can graph it as follows:

1. Given the first line:

`y=12-2.5x`

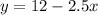
You can identify that it is written in Slope-Intercept Form:

`y=b+mx`

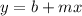
Where "m" is the slope of the line and "b" is the y-intercept.

In this case:

`b=12`

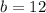
You can find the x-intercept by substituting this value of "y" into the equation and solving for "x":

`y=0`

Because the value of "x" is zero when the line intersects the x-axis.