Question

This is my practice assignment and i will be taking notes on your work!Question 4

Answer 1

Given:

`(y+4)^2-2(y+4)+1`

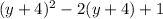
To find:

The factors.

Step-by-step explanation:

i) Let us take,

`t=y+4`

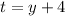
Substituting we get,

`t^2-2t+1`

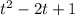
Factorizing we get

`\begin{gathered} t^2-2t+1=t^2-t-t+1 \\ =t(t-1)-(t-1) \\ =(t-1)(t-1) \\ =(t-1)^2 \end{gathered}`

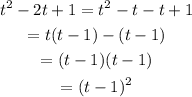
Replace the value of t in the above equation,

`\begin{gathered} =(y+4-1)^2 \\ =(y+3)^2 \end{gathered}`

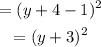
Therefore, the factored form of the polynomial is,

`(y+3)^2`

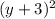
ii) Yes, this is the product of the perfect square trinomial.

So, Teresa is correct.

Final answer:

i) The factored form of the polynomial is,

`(y+3)^2`

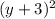
ii) Teresa is correct.