Question

At the end of spring break, Lucy left the beach and drove back towards home, driving at a rate of 60 mph. Lucy's friend left the beach for home 4 hours later, and drove 75 mph. How long did it take Lucy's friend to catch up to Lucy?

Answer 1

Given:

Lucy left the beach and drove back towards home, driving at a rate of 60 mph and Lucy's friend left the beach for home 4 hours later, and drove 75 mph.

To find:

How long it took Lucy's friend to catch up to Lucy.

Solution:

Let Lucy's friend catch up with Lucy in t hours after she starts from the beach.

Since, Lucy started 4 hours before her friend, so she traveled for (t + 4) hours. So, the distance traveled by Lucy is:

`d_1=60(t+4)`

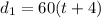
And the distance traveled by Lucy's friend is:

`d_2=75(t)`

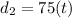
Now, the distances traveled by Lucy and her friend till she catches up to Lucy are equal. SO,

`\begin{gathered} d_1=d_2 \\ 60(t+4)=75t \\ 60t+240=75t \\ 15t=240 \\ t=(240)/(15) \\ t=16 \end{gathered}`

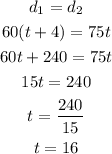
Thus, it took 16 hours to catch up to Lucy.