Question

The following dataset represents the math test scores for a class of 20 students.90, 60, 85, 100, 100, 90, 100, 75, 100,95, 95, 85, 30, 100, 40, 15, 100, 90, 70, 80Identify the best measure of central tendency for this dataset.

Answer 1

The test score of 20 students are :

90, 60, 85, 100, 100, 90, 100, 75, 100,95, 95, 85, 30, 100, 40, 15, 100, 90, 70, 80

Number of students = 20

Mean : The mean (average) of a data set is found by adding all numbers in the data set and then dividing by the number of values in the set.

`\text{ Mean = }\frac{Sum\text{ of all data}}{Total\text{ number of data}}`

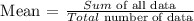
In the given question

Sum of all data = 90+60+85+100+100+90+100+75+100+95+95+85+30+100+40+15+100+90+70+80 = 1600

Sum of all data = 1600

Substitute the value in the expression of Mean :

`\begin{gathered} \text{ Mean = }\frac{Sum\text{ of all data}}{Total\text{ number of data}} \\ \text{Mean}=(1600)/(20) \\ \text{Mean}=80 \end{gathered}`

Mean is 80

Mode : The mode is the number that occurs most often in a data set.

In the given data, frequency of each marks :

In the table, we can see that the maximum number of students has obtained 100 marks so

Mode = 100

Median : Median is the middle value of the set of the order Data

It express as :

`\begin{gathered} \text{Median}=(n+1)/(2)^(th)\text{ when n is odd} \\ \text{Median}=((n+1)/(2)^(th)+(n)/(2)+1^(th))/(2)\text{ when n is even} \end{gathered}`

Arrange the data in the ascending order : 15, 30, 40, 60, 70, 75, 80, 85, 85, 90, 90, 90, 95, 95, 100, 100, 100, 100, 100, 100

n is the total number of terms :

as n = 20, which even so:

`\begin{gathered} Median=\frac{((n)/(2)^(th)+(n)/(2)+1^(th))\text{ Observation}}{2} \\ \text{Median}=\frac{((20)/(2)^(th)+(20)/(2)+1^(th))\text{Observation}}{2} \\ \text{Median}=\frac{(10^(th)+(10+1)^(th))\text{Observation}}{2} \\ \text{Median}=\frac{(10^(th)+11^(th))\text{Observation}}{2} \\ Median=(90+90)/(2) \\ \text{Median}=(180)/(2) \\ \text{Median}=90 \end{gathered}`

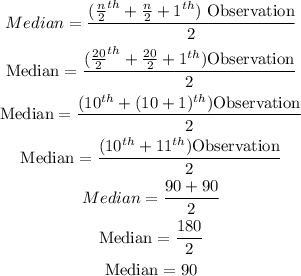
Median is 90

Answer :

The mode 100

The mean 80

The median 90