Question

At the city museum, children’s admission is $5.80 in adult admission is $9.00 on Monday 162 tickets were sold for a total sale of $1176.40 how many children tickets were so that day

Answer 1

Given:

At the city museum, child admission is $5.80 and adult admission is $9.00.

Let the number of children's tickets = x

And the number of the adult tickets = y

on Monday 162 tickets were sold ⇒ x + y = 162

The tickets were sold for a total sale of $1176.40 ⇒ 5.8x + 9y = 1176.40

So, we have the following system of equations:

`\begin{gathered} x+y=162\rightarrow(1) \\ 5.8x+9y=1176.40\rightarrow(2) \end{gathered}`

From equation (1) x = 162 - y

substitute (x) into equation (2)

`5.8(162-y)+9y=1176.40`

Solve the equation to find (y):

`\begin{gathered} 5.8*162-5.8y+9y=1176.4 \\ 939.6+3.2y=1176.4 \\ 3.2y=1176.4-939.6 \\ 3.2y=236.8 \\ \\ y=(236.8)/(3.2)=74 \end{gathered}`

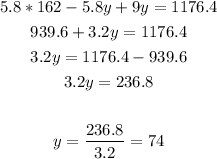
substitute (y) to find (x)

`x=162-74=88`

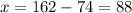
So, the answer will be:

The number of children tickets = 88

The number of adult tickets = 74