SOLUTION
Step 1:
In this question, we are given that a shipping container is in the shape of a right rectangular prism with a length of 9.5 feet, a width of 8.5 feet, and a height of 1.5 feet.
The container is completely filled with contents that weigh, on average, 0.25 pounds per cubic foot.
We are meant to find the weight of the contents in the container, (to the nearest pound).
Step 2 :

Step 3:
Assuming that the container is completely filled with contents that weigh, on average, 0.25 pounds per cubic foot.
The weight of the contents in the container, (to the nearest pound)
=
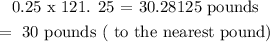
CONCLUSION:
The weight of the contents in the container, (to the nearest pound) =
30 pounds.