The area of a circle is given by the formula
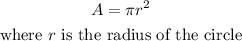
(a) Given that the radius of the circle is 9.2 feet, the area of the circle is.
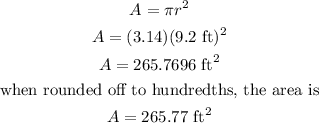
(b) Now that we know the area of the circle, we can now solve for the area of 3 sectors of circle when it is divided by 8.
First, divide the area to 8, then multiply it by 3.
