Question

A cart that weighs 70 N is pushed 50 meters from rest with 1400 N. What is the finalvelocity of thecart?

Answer 1

The weight of cart can be expressed as,

`W=mg`

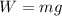
Plug in the known value,

`\begin{gathered} 70N=m(9.8m/s^2) \\ m=\frac{70\text{ N}}{9.8m/s^2}(\frac{1kgm/s^2}{1\text{ N}}) \\ =7.14\text{ kg} \end{gathered}`

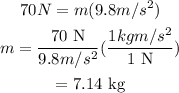
The work done on the cart can be expressed as,

`W=Fd`

According to work energy theorem,

`W=(1)/(2)mv^2-(1)/(2)mu^2`

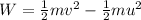
Since the cart is initially at rest therefore, initial speed of cart is zero. Plug in the known expression,

`\begin{gathered} Fd=(1)/(2)mv^2-(1)/(2)m(0)^2 \\ (1)/(2)mv^2=Fd \\ v^2=(2Fd)/(m) \\ v=\sqrt[]{(2Fd)/(m)} \end{gathered}`

Substitute the known values,

`\begin{gathered} v=\sqrt[]{\frac{2(1400\text{ N)(50 m)}}{7.14\text{ kg}}(\frac{1kgm/s^2}{1\text{ N}})} \\ =\sqrt[]{19607.8m^2s^(-2)} \\ \approx140\text{ m/s} \end{gathered}`

Thus, the final velocity of cart is 140 m/s.