Question

Find the percent change and tell whether it is a percent decrease orincreaseOriginal amount: 625End amount: 550

Answer 1

First, we need to find the percentage (P) in his fraction form, which comes from the equation

`550=625\cdot P`

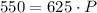
Solving it we get

`P=(550)/(625)=(22)/(25)`

It's our percentage in fraction form... But we need it in his %-form. Converting it is actually easy, we just need to multiply by 100 and % in the end. Doing that ,in this case, leads us