Given data:
* The initial velocity of the box is 0 m/s.
* The final velocity of the box is 2.7 m/s.
* The time taken by the box to reach the final velocity is 30 s.
Solution:
(a). By the kinematics equation, acceleration of the box is,
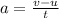
where v is the final velocity, u is the initial velocity, t is the time taken, and a is the accelration,
Substituting the known values,

Thus, the acceleration of the box is 0.09 meter per second squared.
(b). As the acceleration remain uniform,
By the kinematics equation, the distance moved by the box in 6 seconds is,
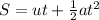
Here t is 6 seconds,
Substituting the known values,

Thus, the distance traveled by the box in 6 seconds is 1.62 m.