The process of multiplying complex numbers is very similar when we multiply two binomials.
The only difference is the introduction of the expression:
![\sqrt[]{-1}\text{ = i}](https://img.qammunity.org/2023/formulas/mathematics/college/ejx6b2k3yrvz8jlz9b9ijvvldmff8rtby5.png)
For example,
Multiply 2i by 8i
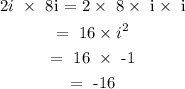
We must remember the following when multiplying imaginary numbers:
![\begin{gathered} \sqrt[]{-1}\text{ = i} \\ i^2\text{ = -1} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/u3gjl8gbnj4l62zu6jl1db5d5qg05eo7vu.png)
Let's work on another example:
(2i)^4:
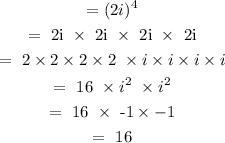
Remember that:

Anytime we raise i to any power, the result changes depending on the value of the power. We can obtain the result by evaluating the terms as shown above.