We know that F varies jointly with q1 and q2 and inversely as the square of d, that means that we can write it as:

where k is a constant. To find k we plug the values given and solve the equation:
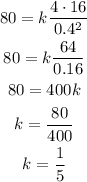
Hence the expression for F is:

Now, plugging the values given we have:

Therefore F=1200.