Answer:
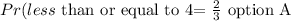
Step-by-step explanation:
Given:
A single die
To find:
the probability of rolling a number less than or equal to 4
The sample space in rolling a single die,= {1, 2, 3, 4, 5, 6}
The sample space for a number less than or equal to 4 = {1, 2, 3, 4}
To get the probability of rolling a number less than or equal to 4, we will apply the formula:
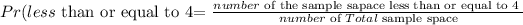
![\begin{gathered} number\text{ of sample space \le4 = 4} \\ number\text{ of total sample space = 6} \\ \\ Pr(less\text{ than or equal to 4\rparen = }(4)/(6) \\ \\ Pr(less\text{ than or equal to 4\rparen= }(2)/(3)\text{ \lparen option A\rparen} \end{gathered}]()