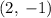Question

Find the vertex of the absolute value function y = 1/2 |x – 2| - 1.(2, -1)(1/2, 2)(2, 1/2)(2, 1)

Answer 1

Given the absolute value function below

`y=(1)/(2)\left|x-2\right|-1`

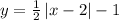
To find the vertex of the absolute value function, we will solve the absolute value function to find the absolute value zero(s) as shown below

`\begin{gathered} |x-2|=0 \\ x=2 \end{gathered}`

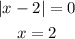
Where, x = 2, substitute 2 for x to find the value of y

`\begin{gathered} y=(1)/(2)|x-2|-1 \\ Where,\text{ }x=2 \\ y=(1)/(2)|2-2|-1=(1)/(2)|0|-1=0-1=-1 \\ y=-1 \end{gathered}`

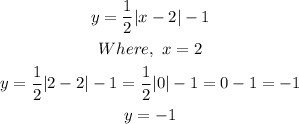
Hence, the vertex absolute value function is

`\left(2,\:-1\right)`