Question

A wooden box of mass 22.5Kg is pulled at uniform velocity along a street surface with a horizontal force of 35.2N. Calculate the coefficient of friction between the box and the street?What leads to an increase in the friction force, an increase in the angle of inclination or a decrease in the angle of inclination?

Answer 1

We need to calculate

Horizontal forces sum

`\sum ^{}_{}F_x=F_{}-Fr=0`

`Fr=F`

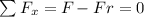
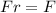
F is the applied force and Fr is the friction force

If F=35.2N, therefore Fr=35.2N

Then for the sum of vertical forces

`\sum ^{}_{}F_y=N-W=0`

`N=W`

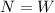
where N is the normal force and W is the weigth

`N=9.8(22.5)=220.5`

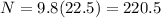
Then for the coefficient, we have

`F_r=\mu N`

`\mu=(Fr)/(N)`

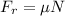
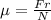
We substitute the values to obtain the coefficient

`\mu=(35.2)/(220.5)=0.16`

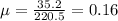
The coefficient of friction is 0.16

Then for the next question

As the angle of inclination increased, the normal force decreased, as we can see in the formula we use previously that will be caused a decrease in the frictional force. Therefore a decrease in the angle of inclination will cause an increase in the friction force.