Question

A gardener has nine identical-looking tulipbulbs, of which each will produce a differentcolor tulip. Six of the colors are unknown,however one will become white, one willbecome yellow, and one will become pink. Heplants them abitrarily in a row. When theflowers start to bloom, what is the probabilitythat the yellow one is first in the row, the whiteone is second, and the pink one is at the end ofthe row?

Answer 1

Given : 9 different colours tulip

white - 1, yellow = 1, pink = 1

(1) Yellow is 1 st in the row

favourable outcome = 1

Total outcome = 9

`P(y)=\frac{\text{favourable outcomes}}{\text{total outcomes}}=(1)/(9)`

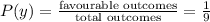
(2) White is 2nd in the row

favourable outcomes = 1

Total outcomes = (9-1) = 8

`P(w)=(1)/(8)`

2) Pink is at the end in the row

favourable outcomes = 1

Total outcomes = (9-2) = 7

`P(w)=(1)/(7)`

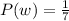
Probability that all 3 are in position

`P(e)=(1)/(9)*(1)/(8)*(1)/(7)=(1)/(504)`

Hence the probability that all three are in position = 1/504