Question

Write an expression in simplest form to represent the area of the shaded region.3r + 2, 2x, 3x, 4x

Answer 1

`\text{Answer : Area is 6x}^2\text{ + 8x OR 2x(3x + 4)}`

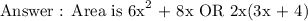
The figure given is a rectangle

The larger rectangle is the outer rectangle while the inner rectangle is the smallest rectangle.

The area of a rectangle is given as

Area = Length x width

Length of the outer rectangle = 3x + 2

The width of the outer rectangle = 4x

The length of the inner rectangle = 2x

The width of the inner rectangle = 3x

The area of the shaded region = Area of the larger rectangle - area of the smaller rectangle

For the larger rectangle

Area = length x width

Area = (3x + 2) x 4x

Open the parenthesis

Area = 4x * 3x + 4x * 2

`\begin{gathered} \text{Area = 12x}^2\text{ + 8x} \\ \text{For smaller rectangle} \\ \text{Area = length x width} \\ \text{Area = 2x }\cdot\text{ 3x} \\ \text{Area = 6x}^2 \\ \text{The area of the shaded region = area of the larger rectangle - area of the smaller rectangle} \\ \text{Area of the shaded portion = 12x}^2+8x-(6x^2\text{)} \\ \text{Area of the shaded region = 12x}^2+8x-6x^2 \\ \text{Collect the like terms} \\ \text{Area = 12x}^2-6x^2\text{ + 8x} \\ \text{Area = 6x}^2\text{ + 8x OR 2x(3x + 4)} \end{gathered}`